
List of prime numbers in order code#
Here is JavaScript code to generate a list of an arbitrarily large number of prime numbers.īecause this is JavaScript, you can even open up your browser's JavaScript console and run this code for yourself.
List of prime numbers in order how to#
How to Create a List of Primes Using the Sieve of Eratosthenes An illustration of how the Sieve of Eratosthenes Works step-by-step. I assembled this list for my own uses as a programmer, and wanted to share it with you.īefore I show you the list, here's how to generate a list of prime numbers of your own using a few popular languages. It may not be intentional on the part of Mother Nature, but prime numbers show up more in nature and our surrounding world than we may think.Here's a list of all 2,262 prime numbers between zero and 20,000. This may sound implausible (obviously, cicadas don't know math), but simulation models of 1,000 years of cicada evolution prove that there is a major advantage for reproductive cycle times based on primes. By using a reproductive cycle with a prime number of years, cicadas would be able to minimize contact with predators. For example, if the cicada evolved towards a 12-year reproductive cycle, predators who reproduce at the 2, 3, 4 and 6 year intervals would find themselves with plenty of cicadas to eat. Any predator reproductive cycle that divides the cicada's cycle evenly means that the predator will hatch out the same time as the cicada at some point. Why this specific number? Scientists theorize that cicadas reproduce in cycles that minimize possible interactions with predators. Cicadas spend most of their time hiding, only reappearing to mate every 13 or 17 years. Two primes are considered as sufficiently secure if they are 2,048 bits long, because the product of these two primes would be about 1,234 decimal digits. The security of this type of cryptography relies on the difficulty of factoring large composite numbers, which is the product of two large prime numbers.Ĭonfidence in modern banking and commerce systems hinges on the assumption that large composite numbers cannot be factored in a short amount of time. Public-key cryptography, or RSA encryption, has simplified secure transactions of all times. This early form of encryption paved the way for Internet security, putting prime numbers at the heart of electronic commerce. In 1978, three researchers discovered a way to scramble and unscramble coded messages using prime numbers. In fact, proof of the Riemann Hypothesis, based on Bernhard Riemann's theory about patterns in prime numbers, carries a $1 million prize from the Clay Mathematics Institute. then count assigned to 0, the inner loop finds the divisors of each j value, count value represents no.of divisors. 3) The for loop iterates from j2 to jgiven number. 2) Read the n value using scanner object sc.nextInt ()and store it in the variable n. The conjectures and theories put out by mathematicians at the time revolutionized math, and some have yet to be proven to this day. 1) We are finding the prime numbers within the limit. all numbers) to find a list of prime numbers exhaustively and quickly. In the 17th century, mathematicians like Fermat, Euler and Gauss began to examine the patterns that exist within prime numbers. A prime number is a natural number whose divisors are 1 and itself (like 2, 3, 5.

This sieve enables someone to come up with large quantities of prime numbers.īut during the Dark Ages, when intellect and science were suppressed, no further work was done with prime numbers.

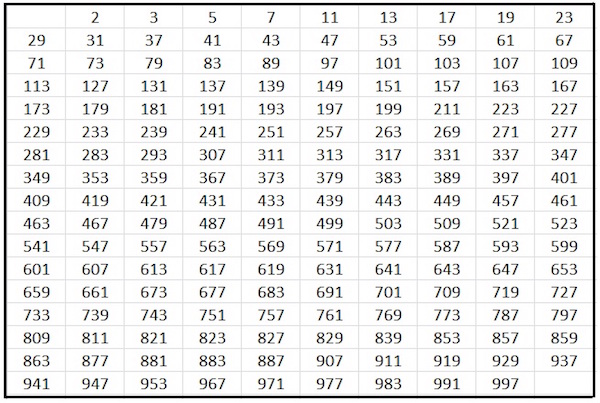
With these multiples crossed out, the only numbers that remain and are not crossed out are prime. So for this chart, you would cross out the multiples of 2, 3, 5 and 7.

Since 6, 8, 9 and 10 are multiples of other numbers, you no longer need to worry about those multiples. For example, with a grid of 1 to 100, you would cross out the multiples of 2, 3, 4, 5, 6, 7, 8, 9, and 10, since 10 is the square root of 100. Eratosthenes put numbers in a grid, and then crossed out all multiples of numbers until the square root of the largest number in the grid is crossed out. This algorithm is one of the earliest algorithms ever written. In 200 B.C., Eratosthenes created an algorithm that calculated prime numbers, known as the Sieve of Eratosthenes. This grid can be used as a Sieve of Eratosthenes if you were to cross out all of the numbers that are multiples of other numbers.


 0 kommentar(er)
0 kommentar(er)
